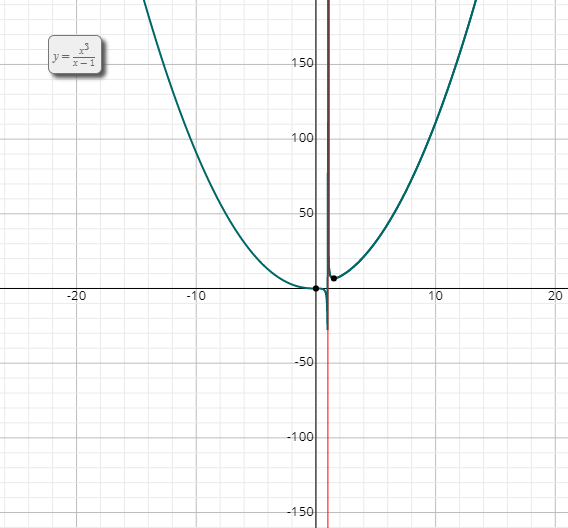
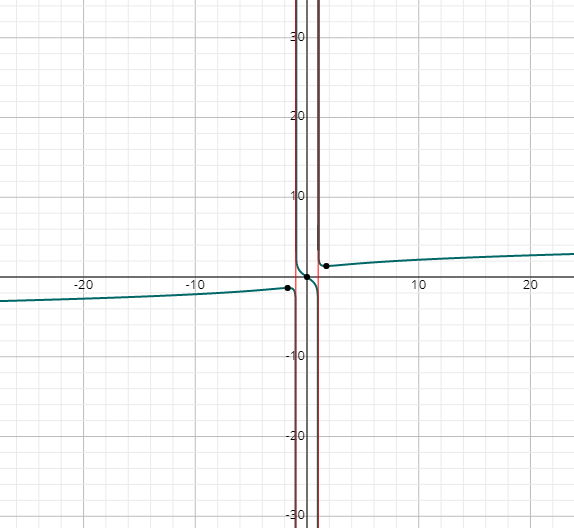
Number 3
D o m a i n o f x 3 x − 1 : [ S o l u t i o n : x < 1 o r x > 1 I n t e r v a l N o t a t i o n : ( − ∞ , 1 ) ∪ ( 1 , ∞ ) ] R a n g e o f x 3 x − 1 : [ S o l u t i o n : − ∞ < f ( x ) < ∞ I n t e r v a l N o t a t i o n : ( − ∞ , ∞ ) ] A x i s i n t e r c e p t i o n p o i n t s o f x 3 x − 1 : X I n t e r c e p t s : ( 0 , 0 ) , Y I n t e r c e p t s : ( 0 , 0 ) A s y m p t o t e s o f x 3 x − 1 : V e r t i c a l : x = 1 E x t r e m e P o i n t s o f x 3 x − 1 : S a d d l e ( 0 , 0 ) , M i n i m u m ( 3 2 , 27 4 ) \mathrm{Domain\:of\:}\:\frac{x^3}{x-1}\::\quad \begin{bmatrix}\mathrm{Solution:}\:&\:x<1\quad \mathrm{or}\quad \:x>1\:\\ \:\mathrm{Interval\:Notation:}&\:\left(-\infty \:,\:1\right)\cup \left(1,\:\infty \:\right)\end{bmatrix}\\
\mathrm{Range\:of\:}\frac{x^3}{x-1}:\quad \begin{bmatrix}\mathrm{Solution:}\:&\:-\infty \:<f\left(x\right)<\infty \\ \:\mathrm{Interval\:Notation:}&\:\left(-\infty \:,\:\infty \:\right)\end{bmatrix}\\
\mathrm{Axis\:interception\:points\:of}\:\frac{x^3}{x-1}:\quad \mathrm{X\:Intercepts}:\:\left(0,\:0\right),\:\mathrm{Y\:Intercepts}:\:\left(0,\:0\right)\\
\mathrm{Asymptotes\:of}\:\frac{x^3}{x-1}:\quad \mathrm{Vertical}:\:x=1\\
\mathrm{Extreme\:Points\:of}\:\frac{x^3}{x-1}:\quad \mathrm{Saddle}\left(0,\:0\right),\:\mathrm{Minimum}\left(\frac{3}{2},\:\frac{27}{4}\right) Domain of x − 1 x 3 : [ Solution : Interval Notation : x < 1 or x > 1 ( − ∞ , 1 ) ∪ ( 1 , ∞ ) ] Range of x − 1 x 3 : [ Solution : Interval Notation : − ∞ < f ( x ) < ∞ ( − ∞ , ∞ ) ] Axis interception points of x − 1 x 3 : X Intercepts : ( 0 , 0 ) , Y Intercepts : ( 0 , 0 ) Asymptotes of x − 1 x 3 : Vertical : x = 1 Extreme Points of x − 1 x 3 : Saddle ( 0 , 0 ) , Minimum ( 2 3 , 4 27 )
Number 4
D o m a i n o f x x 2 − 1 3 : [ S o l u t i o n : x < − 1 o r − 1 < x < 1 o r x > 1 I n t e r v a l N o t a t i o n : ( − ∞ , − 1 ) ∪ ( − 1 , 1 ) ∪ ( 1 , ∞ ) ] R a n g e o f x x 2 − 1 3 : [ S o l u t i o n : f ( x ) > − 1 3 ⋅ ∞ I n t e r v a l N o t a t i o n : ( − 1 3 ⋅ ∞ , ∞ ) ] A x i s i n t e r c e p t i o n p o i n t s o f x x 2 − 1 3 : X I n t e r c e p t s : ( 0 , 0 ) , Y I n t e r c e p t s : ( 0 , 0 ) A s y m p t o t e s o f x x 2 − 1 3 : V e r t i c a l : x = − 1 , x = 1 E x t r e m e P o i n t s o f x x 2 − 1 3 : M a x i m u m ( − 3 , − 3 2 3 ) , M i n i m u m ( 3 , 3 2 3 ) \mathrm{Domain\:of\:}\:\frac{x}{\sqrt[3]{x^2-1}}\::\quad \begin{bmatrix}\mathrm{Solution:}\:&\:x<-1\quad \mathrm{or}\quad \:-1<x<1\quad \mathrm{or}\quad \:x>1\:\\ \:\mathrm{Interval\:Notation:}&\:\left(-\infty \:,\:-1\right)\cup \left(-1,\:1\right)\cup \left(1,\:\infty \:\right)\end{bmatrix}\\
\mathrm{Range\:of\:}\frac{x}{\sqrt[3]{x^2-1}}:\quad \begin{bmatrix}\mathrm{Solution:}\:&\:f\left(x\right)>\sqrt[3]{-1}\cdot \infty \\ \:\mathrm{Interval\:Notation:}&\:\left(\sqrt[3]{-1}\cdot \infty \:,\:\infty \:\right)\end{bmatrix}\\
\mathrm{Axis\:interception\:points\:of}\:\frac{x}{\sqrt[3]{x^2-1}}:\quad \mathrm{X\:Intercepts}:\:\left(0,\:0\right),\:\mathrm{Y\:Intercepts}:\:\left(0,\:0\right)\\
\mathrm{Asymptotes\:of}\:\frac{x}{\sqrt[3]{x^2-1}}:\quad \mathrm{Vertical}:\:x=-1,\:x=1\\
\mathrm{Extreme\:Points\:of}\:\frac{x}{\sqrt[3]{x^2-1}}:\quad \mathrm{Maximum}\left(-\sqrt{3},\:-\frac{\sqrt{3}}{\sqrt[3]{2}}\right),\:\mathrm{Minimum}\left(\sqrt{3},\:\frac{\sqrt{3}}{\sqrt[3]{2}}\right) Domain of 3 x 2 − 1 x : [ Solution : Interval Notation : x < − 1 or − 1 < x < 1 or x > 1 ( − ∞ , − 1 ) ∪ ( − 1 , 1 ) ∪ ( 1 , ∞ ) ] Range of 3 x 2 − 1 x : [ Solution : Interval Notation : f ( x ) > 3 − 1 ⋅ ∞ ( 3 − 1 ⋅ ∞ , ∞ ) ] Axis interception points of 3 x 2 − 1 x : X Intercepts : ( 0 , 0 ) , Y Intercepts : ( 0 , 0 ) Asymptotes of 3 x 2 − 1 x : Vertical : x = − 1 , x = 1 Extreme Points of 3 x 2 − 1 x : Maximum ( − 3 , − 3 2 3 ) , Minimum ( 3 , 3 2 3 )
#340153 on Dec 2023
Comments