Answer to Question #174473 in Discrete Mathematics for boateng

Question

In a class of 32 students, 18 offers chemistry, 16 offers Physics 22 offer Mathematics. 6 offer all three subjects, 3 offer Chemistry and Physics only and 5 offer only physics only. Each student offers at least one subject. Find the number of student who offer A. chemistry only B. only one student C. only two subjects

Answer 1

$$N(P\cup C\cup M)=32$$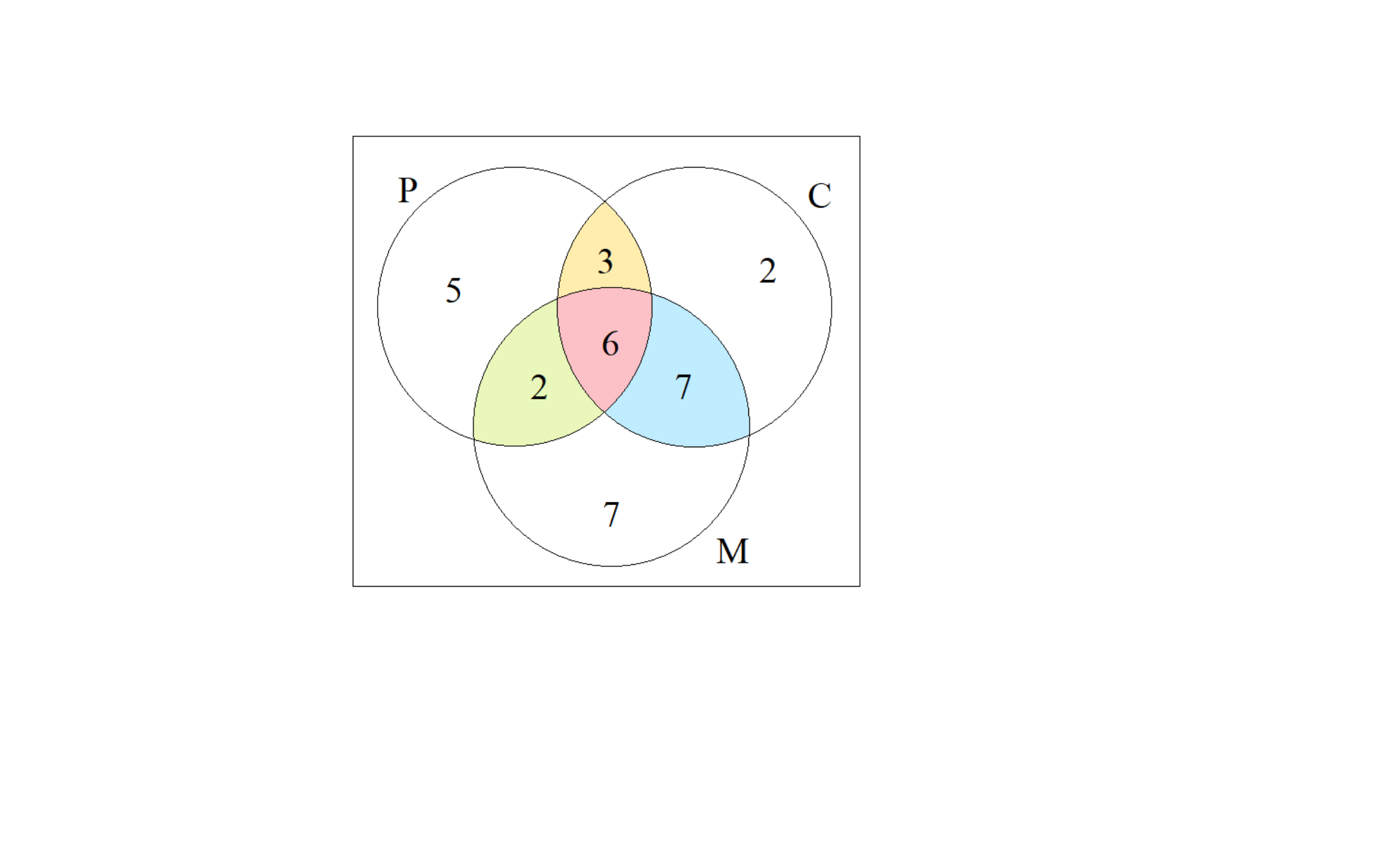

$$N(P)=16, P(C)=18, P(M)=22$$

$$N(P\cap C\cap M)=6$$

$$N(P\cap C \cap M^C)=3$$

$$N(P\cap C^C \cap M^C)=5$$

$$N(P\cap M \cap C^C)=N(P)-N(P\cap C\cap M)-$$

$$-N(P\cap C \cap M^C)-N(P\cap C^C \cap M^C)$$

$$=16-6-3-5=2$$

$$N(P\cup C\cup M)=N(P)+N(M)+N(C)$$

$$-N(P\cap M \cap C^C)-N(P\cap C \cap M^C)-$$

$$-N(C\cap M \cap P^C)-2N(P\cap C\cap M)$$

$$N(C\cap M \cap P^C)=16+18+22$$

$$-32-3-2-2(6)=7$$

A. $N(C\cap P^C\cap M^C)=2$

B. $N(\text{only one subject})=5+2+7=14$

C. $N(\text{only two subjects})=3+2+7=12$