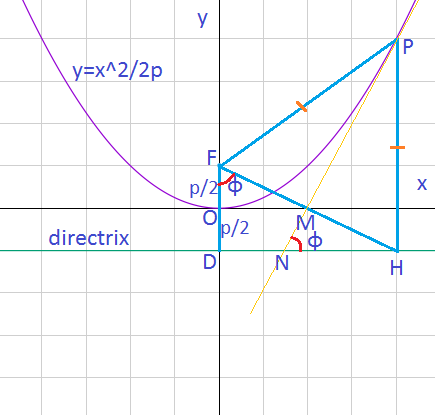
1. Let F be the focus of the parabola x2=2py, DH be its directrix, FO=OD=2FD =2p. From the definition of parabola it follows that PF=PH, where P is any point on the parabola and H its projection on the directrix. −−−−−−−−−−−−−−−−−−−−−−2. The slope of the tangent at the point P is determined by the formula:k=tan(φ)=y′(x)=(2px2)′=px.Because tan(∠DFH) = DFDH =p x = tan(φ), then ∠DFH=φ.−−−−−−−−−−−−−−−−−−−−−−3. Consider triangles FDH and NMH. The angle H is common to them, ∠DFH=∠MNH as shown above, so the angle NMH is right:∠NMH=∠FDH=90°.−−−−−−−−−−−−−−−−−−−−−−4. In the isosceles triangle FPH the height PM is the median: FM=MH, so the point M lies on the midline OM of the triangle FDH, that is, on tangent OM of the parabola at its vertex. It is obvious that the set of points M represent the tangent at its vertex, what we wanted to prove.


Comments